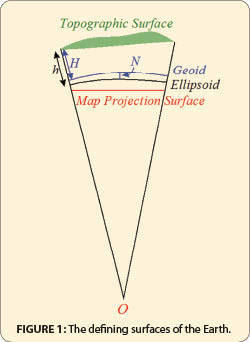
The Earth is composed of several physical and mathematical surfaces that play important roles in surveying. As shown in green in Figure 1, the surface that we are most familiar with is the topographic surface. This is the top layer of the Earth that most people spend their entire life on. It is the surface on which property surveys are performed, maps are created, and construction surveys modified.
In addition to human changes to this surface, the plates that compose this surface are in constant motion. Most of the United States is moving in a southwesterly direction. The collision of two different plates is causing the Rocky Mountains to continue to rise, and their drifting is causing splits in the surfaces such as the Baja California Peninsula.
Thus, surveyors on the West Coast of the United States must take the published coordinates for control monumentation and determine their location based on the velocity vectors of these plates. For surveyors on the East Coast, velocity vectors of the control coordinates are relatively small and are usually ignored.
However, it has been determined that the East Coast experiences a major earthquake once every 200 years. The last major earthquake for the East Coast was in 1814, so the East Coast is due for one in the upcoming years. How big will this earthquake be? Well, the East Coast is on a portion of the oldest plate on the Earth, and thus it is the most rigid. During the 1814 earthquake, the Mississippi River flowed backwards for three days and glass was broken in Philadelphia.
The East Coast has been experiencing many minor tremors over the past recent years. In 2012, the 5.8-magnitude earthquake in Virginia was a reminder that the East Coast is tectonically active. When the next major earthquake does occur, many of our control monuments will have incorrect coordinates for their new positions. Even without this earthquake, a look at the positions of Continuously Operating Reference Stations (CORS) such as WIL1 tells us that we are slowly moving westward. This motion is not great, and without fault lines neighboring points are most likely moving at about the same rate. However, where fault lines do exist, monuments on one side of a fault line shift with respect to those on the other side, and thus this motion is important to consider. This all explains why the National Geodetic Survey decided to stop supporting most monumented control.
The Geoid
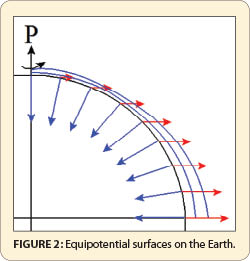The entire Earth is composed of an infinite number of gravitational surfaces. These surfaces are defined by their potential to do work and are known as equipotential surfaces; that is, these are surfaces that have an equal ability to do work. Work is defined as force times distance. In the case of the equipotential surfaces, the gravitational attraction applies the force, and the distance is defined as the distance of a point from the mass center of the Earth.
However, as we all know, the Earth rotates once a day. The centrifugal force caused by this rotation, shown as red arrows in Figure 2, works against the pull of gravity, which is shown as blue arrows. It is greatest at the equator and goes to zero at the pole, P. Additionally, as given by Newton’s universal law of gravitation, the force of gravity decreases with increasing distance between two objects.
Because the equatorial axis of the Earth is longer than the polar axis of the Earth, the force of gravity is greater at the poles than at the equator. This combined effect of the varying distances from the mass center of the Earth and the rotation of the Earth means that the force of gravity is less at the Equator than at either pole.
Because equipotential surfaces are defined by both force and distance, the distance between the equipotential surfaces must decrease as the force of gravity increases. Thus, the equipotential surfaces converge at the poles, which means they are closer at the poles than at the equator, as shown with the blue lines. These surfaces also undulate as the moon passes over the Earth and as densities of earth change in localities. (Instead of dieting you could simply go to the equator and stand on the highest mountain if you wish to weigh less! Mount Chimborazo in Ecuador might be a good choice.)
In surveying, one equipotential surface is arbitrarily assigned a value of zero, which defines the geoid. All other equipotential surfaces are then referred to by their height above or below the geoid. In the National Geodetic Vertical Datum of 1929 (NGVD 29), the geoid was defined by the average height of 26 tide gauge stations that were located on the Atlantic, Pacific, and Gulf coasts. Thus elevations were said to be referenced to sea level.
However, sea levels change with time, so the North American Vertical Datum of 1988 (NAVD 88) was referenced to one bench mark at the mouth of the St. Lawrence Seaway, called Father Point, in Rimouski, Quebec, Canada. Thus, the equipotential surface that passes through a specific bench mark at Father Point was assigned a height, and all other bench marks in the NAVD 88 are relative to this single bench mark. The assignment of this height at Father Point then determined where the zero equipotential surface was and, thus, the geoid that establishes NAVD 88.
The height of a point above the geoid is known as its orthometric height or commonly known as elevation, which is designated by H. However, from the preceding, it is obviously incorrect to refer to the elevation of a point as “referenced to mean sea level” because the new datum is only related to a single bench mark.
The Ellipsoid
Another surface that plays a major role in surveying is a mathematical surface that best fits the geoid. This surface, known as the ellipsoid, was traditionally defined to fit the geoid in a particular region. For example, the Clarke 1866 ellipsoid was defined to fit the geoid on the African continent. I am not a geologist so am guessing here, but possibly because the North American plate and the African plate were once connected, this ellipsoid fit the geoid closely for the North American plate also.
However, with the advent of satellites, it was obvious that a global ellipsoid was needed. These ellipsoids are designed to best fit the geoid for the entire Earth. The North American Datum of 1983 (NAD 83) and the International Reference Systems use the Geodetic Reference System of 1980 (GRS 80). However, the Department of Defense (DoD) decided to use the World Geodetic System of 1984 (WGS 84). The difference in the shape of these two ellipsoids is minimal with a slight difference in the flattening factor. Again, the ellipsoids determine only the mathematical figure that approximates the geoid. That is used to determine the coordinates of points but does not determine these coordinates. It is how the ellipsoid is placed on the geoid that determines coordinates.
Datum
A datum is also called a reference frame because it is a framework of stations with coordinates related to the particular placement of the ellipsoid on the geoid. For example, modern reference frames are defined by the orientation of the polar axis and the position of the mass center of the Earth.
However, due to the tilt of the Earth with respect to its orbital plane, which is about 23.4°, the pull on the two hemispheres of the Earth by the Sun is not uniform. This causes the Earth to wobble much like a child’s top. This wobble means that the polar axis of the Earth is always on the move. (It was the fact that the pole was moving into the zodiac cluster known as Aquarius that led to the 1960s song “Age of Aquarius.”)
Thus, by international convention, the polar axis of the Earth was defined by the mean positions of the pole between 1900.0 and 1905.0, which is designated as the Conventional Terrestrial Pole (CTP). As shown in Figure 3, the CTP defines the Z axis of the Earth with the mass center of the Earth defining the coordinate system’s origin.
The X axis lies in the equatorial plane and is defined by the Greenwich meridian, which originally passed through the vertical axis of a telescope at the Royal Observatory in Greenwich, England but has since moved about 200 feet to the east. The Y axis, which also lies in the equatorial plane of the Earth, creates a right-handed coordinate system for the entire Earth.
This XYZ coordinate system is the basis for GPS baseline vectors and is known as a geocentric coordinate system. It is also sometimes called a topocentric coordinate system. When the datum is referenced to a coordinate system that has its origin at the mass center of the Earth and its orientation aligned with the Earth’s polar axis, it is known as an earth-centered, earth-fixed (ECEF) coordinate system.
A reference frame is a system of stations whose positions are precisely defined with respect to the placement of the ellipsoid. Over time we have gotten much better observations to determine the mass center and spin axis of the Earth. This has resulted in new definitions for our datum; that is, the ellipsoid has been slightly shifted and/or rotated. For example, the DoD changed the WGS 84 datum four times since its initial inception. This means coordinates that defined the position of a station in 2011 are different than those that are observed today with the same GPS receiver. Again, an ellipsoid is simply a mathematical shape that approximates the shape of the geoid. A horizontal datum or reference frame is a system of stations with coordinates that position the ellipsoid with respect to the geoid.
Similarly, NAD 83 was defined when GPS was in its infancy. Due to much better knowledge about the Earth, which was generated by GPS and other technologies (discussed later), NAD 83 has also been improved many times. In this process, the NGS realized that it was hopeless to continuously update the coordinates of the original monumentation, which is not crucial to the industry because GPS could bring precise coordinates to most locals within minutes. Thus, the monumentation that was defined by conventional surveys, known as the legacy system, is not being updated with new coordinates.
Only High Accuracy Reference Network (HARN) and CORS coordinates are being updated in what is currently known as NAD 83(2011) (epoch 2010.0). (The time stamp of 2010 should now make sense to the reader because this means the coordinates as of January 1, 2010 at 0 hr.) Thus, the legacy system of monuments and NAD83 (2011) are two different coordinate systems and should not be mixed in a survey. However, there are mathematical procedures that can be used to bring one coordinate system into the other. This is the topic for a later article.
The reader should realize that neither the stations nor the ellipsoids have changed but rather the coordinates of the stations, and thus the positions of the ellipsoids with respect to the geoid. What this means is that the coordinates of a point observed before the coordinate refinement in 2012 by GPS will be different than the most current coordinates. This is similar to but not as drastic as what happened when NAD 83 replaced the North American Datum of 1927 (NAD 27).
The Geodetic Coordinate System
As shown in Figure 4, the ellipsoid is further broken into lines running north and south, known as meridians, and parallels of latitude running east and west. This angular system of lines, which are normally drawn on a globe, creates the basis for the geodetic coordinate system.
The plane that is perpendicular to the semiminor axis (polar axis) of the ellipse and passes through the center of the ellipse defines the equatorial plane. The plane passing through a location in Greenwich, England defines the Greenwich Meridian. The Greenwich Meridian is defined as zero degrees longitude, and all other meridians are located by their angular distance from the Greenwich Meridian. Similarly, the equator is defined as zero degrees latitude, and all other latitudes are based off the angular distance from the equator.
Using these definitions, all locations on the Earth can be determined by their angular values from these two defining lines and the height above the ellipsoid h. For example, point P shown in Figure 5 is located by the angular values of latitude φ, longitude λ, and height h. These three coordinate values are the coordinates for points in what is known as the geodetic coordinate system.
Notice that because the ellipsoid is not a sphere, the radial line passing through P, which defines the curvature of the ellipsoid, does not typically intersect the spin axis of the Earth at the center of the ellipsoid. Furthermore, it is important to recognize here that the geodetic height of a point h is not the equivalent of its orthometric height H because h is related to the ellipsoid and H is related to the geoid.
The difference in these heights is defined by the amount of separation between the geoid and the ellipsoid below a point, which is shown in Figure 1 and designated as geoid height, N. This relationship is mathematically expressed as h = H + N where h is the geodetic height of a point, H its orthometric height (elevation), and N the geoid height. (This is not a truly equivalent equation because H follows the direction of gravity from P, which follows a curved line to the geoid, and h is along a line perpendicular to the ellipsoid. However, this difference is small and is usually ignored.)
Refinement of Horizontal Coordinate Systems
The ellipsoids form the computational basis for our horizontal coordinate systems. A coordinate system, which is also called datum and reference frame, is composed of monuments that are interconnected by observations to determine their relative positions with respect to the position of the coordinate system’s origin and orientation. In NAD 27 the datum was defined relative to a single point in Kansas known as Meades Ranch and the azimuth to another station called Waldo. NAD 27 was not an ECEF coordinate system.
During the latter half of the 20th century technologies were created to determine the relative position of points on different continents of the Earth. These technologies include very long baseline interferometry (VLBI), satellite laser ranging (SLR), lunar laser ranging (LLR), doppler orbitography and radio positioning integrated by satellite (DORIS), and GPS. With these technologies, it was now possible to connect continents with extremely high accuracy and create a reference system that ties the entire Earth together—thus, the creations of the ECEF coordinate systems.
As previously discussed, to create a coordinate system that could work in a global sense, it was decided that the NAD 83 datum should have its origin defined by the mass center of the Earth, with its Z-axis (orientation) defined by the spin axis of the Earth. This system, like other ECEF coordinate systems, shares this common definition for positioning the ellipsoid.
In 1987 the International Earth Rotation and Reference System Service (IERS) was founded. Since that time, they have developed new realizations of coordinate systems and have published these as ITRF89, ITRF90, ITRF91, ITRF92, ITRF93, ITRF94, ITRF95, ITRF96, ITRF97, ITRF2000, ITRF2005, and ITRF2008. (The definition of these coordinate systems can be found at www.iers.org/nn_10968/IERS/EN/DataProducts/ITRF/itrf.html?__nnn=true.)
They also publish the position of the Earth’s “instantaneous” pole in relation to the Conventional Terrestrial Pole (CTP). These reference frames are known as International Terrestrial Reference Frames (ITRF), which provide our most current and accurate positions for points on the Earth as well as the mass center of the Earth.
How does this affect us? Well, originally the WGS 84 and NAD 83 were considered to be the same because the differences in the two ellipsoids were minor. However, the Department of Defense has redefined the WGS 84 datum (coordinate system) four times to agree with various International Terrestrial Reference Frames. Thus, the origin for the original NAD 83 datum, the legacy system, is now off by about 5 feet from the currently accepted location for the mass center of the Earth. This means that GPS coordinates determined by the broadcast ephemeris will not agree with coordinates of legacy NAD 83 control monuments.
In 2007 the NGS published new coordinates for all CORS and HARNS with respect to ITRF 2000 when creating the National Spatial Reference System (NSRS) 2007. This was done again in 2011. This means that if we are to use GPS and have legacy coordinates, we must “localize” the GPS-derived coordinates to our monumented system of coordinates.
Map Projection Coordinate Systems
The final surface that surveyors use is a map projection coordinate system shown in Figure 5, which defines, for example, the state plane coordinate system for each zone. This surface is probably the easiest system available for everyday use. However, as is evident from Figure 1, a map projection system must distort some curvilinear features to create a plane projection of the points. In the case of the three map projection systems used in state plane coordinates, the observation that is distorted is distance. This is the topic for my next article.
If we are to observe orthometric heights then we must know not only the difference in elevation but also the force of gravity along our level lines. If we are to work in geodetic coordinates then we must be prepared to work in the curvilinear geodetic coordinate system shown in Figures 4 and 5 or the geocentric coordinate system shown in Figure 3. If we are to work in state plane coordinates then we must reduce our distances, because, as shown in Figure 1, the distance observed at the surface will not match the distance on the mapping surface.
All of these systems are related, and one can go between these systems using a coordinate transformation processes. All of this is the subject of later articles. This article lays the foundation for the coordinate systems. It defines the terms that will be used in subsequent articles. Until then, happy surveying.
